Flow Feature Identification in Unsteady Separated Flows

Very complex flow structures occur during separation that can appear in a wide variety of applications involving flow over a bluff body. The ability to detect discrete flow structures in fluid flow environments is of growing interest to a wide variety of applications. For instance, large scale flow structures such as swirling, high shear regions and vortical structures are thought to be controlling mechanisms for chaotic mixing, unsteady pressure fields that influence fluid-surface interactions, transport in multiphase flows, and a host of other applications. A robust means of developing an understanding of how these flow structures develop, evolve, decay, and interact is of fundamental importance. The following work was performed in collaboration with research groups led by Prof. James Liburdy and Dr. Eugene Zhang, and was an outcome of a new interdisciplinary graduate level course on Flow Feature Identification and Scientific Visualization at OSU.

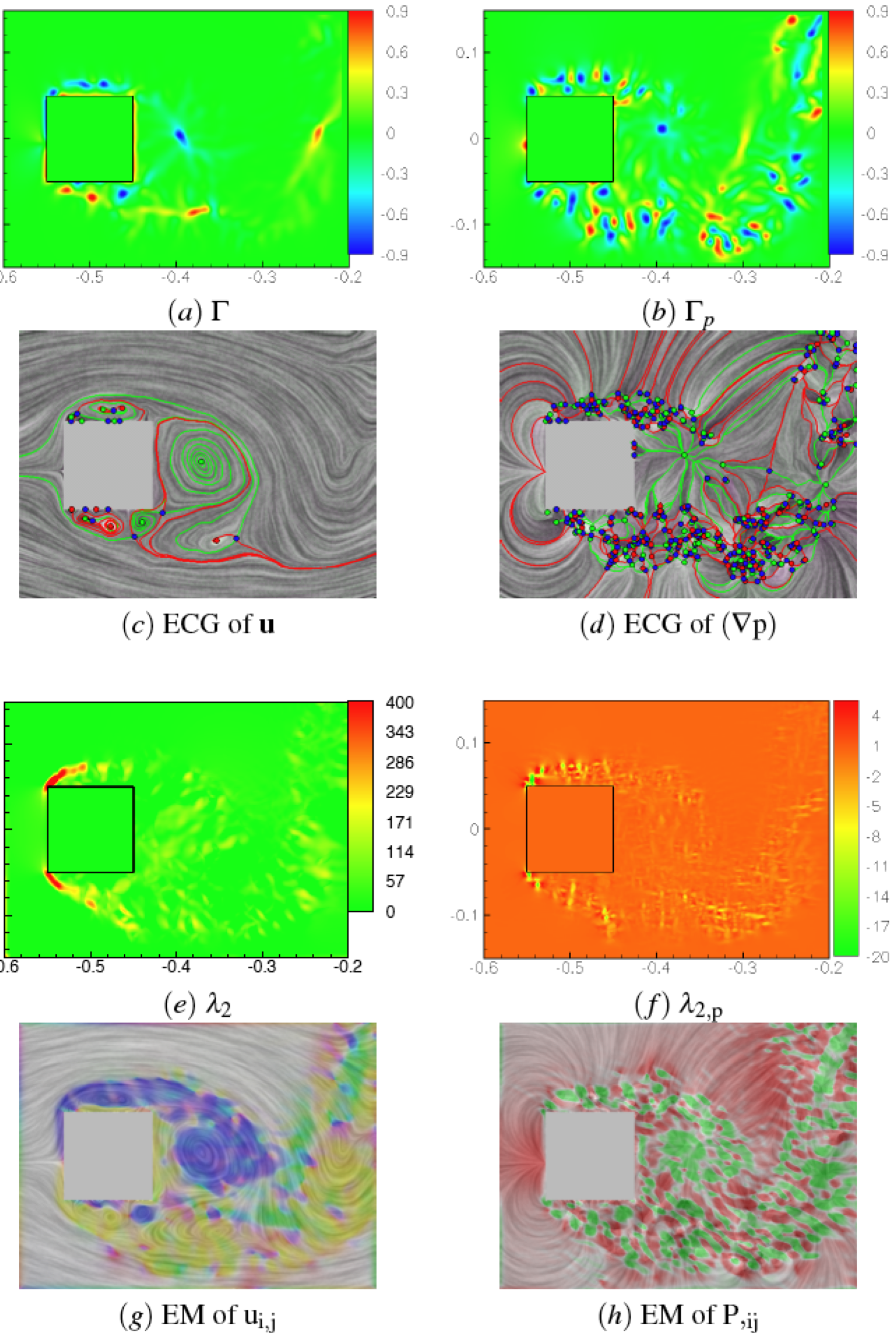We have investigated different flow feature identification techniques based on the vector field (velocity or pressure gradient) and tensor field (velocity gradient or Hessian of pressure) topologies for unsteady separated flow over bluff bodies. Such flows are associated with the Kelvin-Helmholtz shearing instability that results in a roll-up along a highly concentrated vortex sheet. Typically, experimental data (based on PIV for example) are limited to the spatio-temporal distributions of velocity field, wheareas direct numerical simulations provide comprehensive data for velocity as well as pressure fields. Defining feature identifiers based on the Gamma function (vector topology) and the lambda-2 (tensor topology) and using both velocity and pressure fields, we have identified the importance of each approach for flow over an airfoil at 20 degree angle of attack, and flow over a square cylinder for Reynolds numbers on the order of 10,000. It was found that the tensor-based topology (lambda-2 method) usually capture vortical structures on the small scales, whereas the extent of the vortices and large-scale features were better captured by vector-field topology (Gamma function). The Gamma function (based on velocity or pressure gradient) was also able to provide information on the strength associated with a vortical structure. The tensor-based topology (lambda-2 method) usually capture vortical structures on the small scales, whereas the extent of the vortices and large-scale features were better captured by vector-field topology. We have also used techniques developed in the scientific visualization community for analysis of vector and tensor field toplogies to observe similar trends.

The above figures show comparison of the velocity and pressure-gradient based flow descriptors for flow over a square cylinder simulated using direct numerical simulation. Closeup view of the symmetry plane is shown. ECG stands for the vector field topology and includes the streamlines together with flow singularities: sources (green dots), sinks (red dots), saddles (blue dots), and the separatrices or the lines connecting the singularities (green line connects a saddle to a source or repeller, and red line connects the saddle to a sink or attractor). EM stands for the eigenvalue manifold based on the tensor fields (velocity gradient or the pressure Hessian). The velocity gradient eigenvalue manifold shows clockwise rotation (blue) and counterclockwise rotation (yellow) regions on the top and bottom surfaces of the cylinder (Chen et al., IJNAM 2008). Recently we have used these techniques to link the dynamics of vortical structures past a bluff body and the surface pressure oscillations to better identify regions on the surface of the body that are most sensitive to passage of the vortices. Such information and analysis techniques may be important to identify sensor locations for developing flow control strategies.

• Chen, G., Lin, Z, Morse, D., Snider, S., Apte, S.V., Liburdy, J.A., and Zhang, E., 2008, Multiscale feature detection in unsteady separated flows, International Journal of Numerical Analysis and Modeling, Vol. 5, Suppl., pp. 17-35. A special issue on Modeling, Analysis, and Simulations of Multiscale Nonlinear Systems. (PDF)

• Snider, S., Morse, D., Chen, G, Apte, S.V., Liburdy, J.A., and Zhang, E., Detection and analysis of separated flow induced vortical structures, 44th AIAA Aerospace Sciences Meeting, Reno, NV, January 2008. (PDF)

• Snider, S., Morse, D., Apte, S.V., and Liburdy, J., Correlation of surface pressure and vortical flow structures in an unsteady separating flow, 38th AIAA Fluid Dynamics Conference and Exhibit, AIAA-2008-4051, Seattle, WA, June 2008. (PDF)

• Snider, S., 2008, Detection and analysis of separated flow induced vortical structures, M.S. Thesis, School of Mechanical Industrial and Manufacturing Engineering, Oregon State University, Corvallis, OR. (PDF)

• Snider, S., Morse, D., Apte, S.V., and Liburdy, J. Correlations between surface pressure variations and passage of vortical flow structures in separated flow over bluff bodies, in preparation.